Trouton’s rule is the empirical rule for estimating entropy changes of vaporization by using boiling point. The modification of this rule is Hildebrand Rule. As the enthalpies of vaporization and boiling point temperature can changes very significantly for different liquids. Trouton’s rule is a rough approximation. This rule is useful for obtaining the approximate value of heat of vaporization by knowing the boiling point.
What is the enthalpy of vaporization?
The enthalpy of vaporization is the amount of heat added to a liquid to change it into gas. It is also known as latent heat of vaporization or heat of evaporation. It is represented by ∆Hvap.
What is the entropy of vaporization?
The entropy of vaporization is the increase in entropy upon vaporization of the liquid.
Trouton’s Rule:
Discovery:
Frederick Thomas Trouton an Irish physicist stated or formulated the trouton’s rule.

This rule is named after Frederick Thomas Trouton.
Statement:
This rule states that the entropy of vaporization is approximately 85Jmol-1 K-1 for liquids (not all liquids). This is the empirical rule for estimating the entropy of vaporization of liquids by using boiling point.
( ∆S)vap ~ 85Jmol-1 K-1
Entropy change of Benzene and Nitrogen:
As enthalpies of vaporization of liquids vary significantly.
Benzene boils at 353.25 K with ∆Hvap =+30,800 J/mol .It gives entropy increase of
∆S= ∆Hvap /Tb.p
=+30,800/353.25
=87.2 Jmol-1 K-1
And we also see the entropy change of Nitrogen that boils at 77.4 K and ∆Hvap =5818J/mol
∆S= ∆Hvap /Tb.p
∆S= 5818/77.4
=75.2 Jmol-1 K-1
The entropy of carbon tetra-chloride:
The entropy of vaporization of carbon tetrachloride is 29.665J/mol. It is comparable to an experimentally measured value that is30000 J/mol.
The boiling point of CCl4 is 349.9K.
∆S= ∆Hvap /Tb.p
=29.665/349.9
=84.7 Jmol-1 K-1
Entropy change of gases:
As gases occupy more volume than liquids. Entropy is a measure of randomness. In the case of gases, each molecule of gases has many locations. Ammonia and water have hydrogen bonding in their structures. Entropy will increase when the hydrogen-bonded liquid structure is destroyed on vaporization. Both water and ammonia have higher values of entropies because of hydrogen bonding.
Estimation of entropy by changing volume:
If entropy increases on vaporization due to increasing vapor volume. We can also estimate the entropy by using volume change on vaporization:
∆S=Rln(Vvap /Vliq )
For example:
A mole of liquid occupies about 2mL, and the same gas at 25℃ occupies 30L(30,000mL).
∆S=R ln(Vvap /Vliq )
=8.31 ln (30,000/2)
=80 Jmol-1 K-1
This value is very comparable to Trouton’s rule (85 Jmol-1 K-1 ).
Entropy change of liquid and solids:
Solid and liquids are denser than gases and there is a strong interaction between molecules. Phase transition between a liquid and solid and solid and solid create a change in crystal structures and the interaction of molecules. And there is a significant change of entropy for these phase transitions. These liquid-solid and solid-solid phase transitions do not follow trouton’s rule.
There is no general rule for entropies of fusion of solids at melting temperature. For most solids entropy of fusion is very less than the entropy of vaporization.
This also shows that increase in disorder in changing from liquid to vapor is greater than the increase in changing from solid to liquid.
Trouton’s Rule applies to normal liquids. However, there’s a deviation from trouton’s rule as shown in the figure.
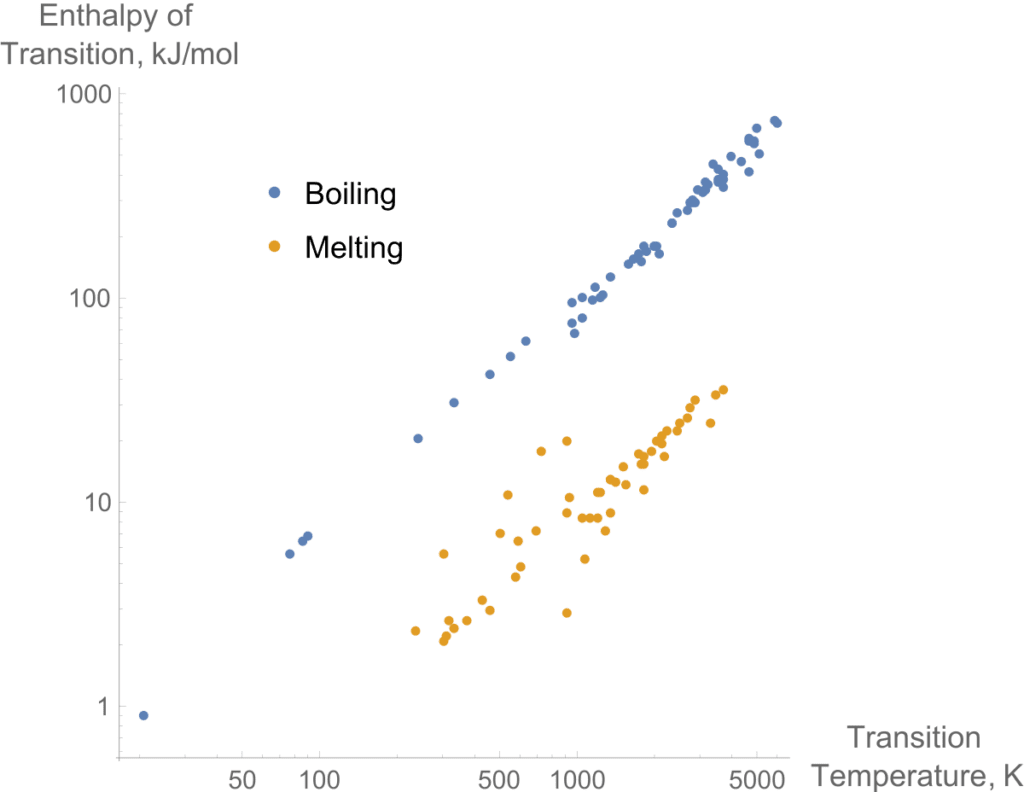
It is clear that normal liquids have universal values but very linearly.
Negative deviance occurs due to the small gas phase. Mostly in small molecules.Such as in methane molecules.
Significance :
Trouton’s rule helps to find the approximate value of heat of vaporization of liquids by knowing the boiling point of the liquid.
The significance of this rule is ( ∆S)vap is always approximately 85Jmol-1 K-1 for liquids but not for all, increase in molecular disorder on vaporization is almost the same for many liquids.
Limitations:
Here are some limitations of this empirical rule;
- This rule fails for substances with boiling point 150K or below
- This rule fails for liquids such as water, alcohol, amines, and ammonia. such liquids are called abnormal liquids.
- For O2 constant of this rule varies from about 75.312 of boiling point at 90 K.
- And a variation of constant for zinc is 97.236 at a boiling point of 1180K.
Trouton’s Rule not applicable to structured liquids:
- The entropies of vaporization of water, ethanol, formic acid, and hydrogen fluoride are different from estimated values.
- If liquids contain hydrogen bonding or any other ordered structure their entropies are low and gain in entropy during vaporization is high. So enthalpy of vaporization of hydrogen-bonded molecules is higher than plane alkanes.
Trouton’s Rule not applicable to order gases:
- The entropy of vaporization of formic acid shows negative deviance from trouton’s rule. This shows the orderly structure in the gas phase. And formic acid forms a cyclic dimer structure in the gas phase. The dimerization in formic acid reduces the entropy of the gas.

- The dotted lines show the hydrogen bonding in the structure of formic acid.
- In the case of water hydrogen bonding show structure phase and reduced entropy of liquid.Water show positive deviation from trouton’s rule.
Modification of Trouton’s Rule: Hilderband’s Rule.
Trouton’s rule is modified by Hilderband.
Hilderband argued that taking as a comparison it would be appropriate to evaluate ( ∆S)vap for a change in the state so the molar volume of different vapors are always the same rather than comparing with the pressure of 0.1 MPa.
He found that for any liquid ‘T’is boiling temperature at which vapors occupies 22.4 L . Then change in entropy of vaporization of liquid may be expressed empirically as;
[ ( ∆S)vap ]T‘ = ( ∆H)vap /T’ =84.935J/℃.
T’ can be found by following conditions;
- Vapour pressure must satisfy the relation
P’= ( Pvap )P’ .
2.On assumption vapors behaving like ideal gas P’ must satisfy the relation
22.4P’=RT’
Above two conditions clear the specificity of temperature T’
The difference between ( ∆H)vap of boiling temperature Tb and T’ is minor so we neglect it.
Entropy unit:
The entropy unit is abbreviated as eU instead J ℃ -1 mol-1.
The eU stands for entropy unit.
1eU=1J ℃ -1 mol-1 .
For calculating a change in entropy:
The entropy is a function of T and P.so to calculate the change in entropy we use the following equation;
∆S=∫_T1^T2 CP dT/T -R∫_P2^P1 dP/P.
Problem:
Cadmium vapours behaves as ideal monoatomic gas,CP =CV +R and CV +3/2R.
CP =5/2R =20.785JK-1 mol-1 .
Given:
T1 =1040K
T2 =1300K
P1 =0.1 MPa.
P2 =0.6 MPa.
Solution :
Put these values in above equation…
∆ S=∫_1300k^1040k 20.785 /T dT + 8.314 ln (1/6)
=-10.330 JK-1 mol-1
Problem:
Calculate entropy change when 1 mol of ice at 0 ℃ is heated to steam at 100 ℃ at 0.1 MPa pressure. Molar heat of fusion of ice at 0 ℃ is 6.008kJ and heat of vaporization of water at 100 ℃ is 40.668kJ. The specific heat of water is approximately 4.184 J℃-1 g-1.
Solution:
Total entropy change is equal to the sum of molar entropies of fusion of ice and vaporization of water with an increase in the entropy between 0 ℃ and 100 ℃.
AT 0 ℃ :
( ∆S)f =6.008/273
=22.007 J℃-1 g-1 .
Again at 100 ℃ :
( ∆ S)vap =40.668/373
=109.029 J℃-1 g-1 .
The increase in entropy of water between 0 ℃ and 100 ℃ .
By using equation..
(∆S)p =∫_T1^T2 CP dT/T =CP ln (T2 /T1 )
The molar heat of water at constant pressure is equal to its molecular weight multiply by its specific heat.
Hence: 18×1=18.
So;
( ∆ S)p =18 × ln (373/273) × 4.184
=18 × 4.184 × 2.303 log (373/273)
=98.383 J℃-1 g-1.
Total entropy change:
= ( ∆ S)vap +( ∆S)f+ ( ∆ S)p
=109.029+22.007+98.383
=229.419 J℃-1 g-1 .
Conclusion:
Trouton’s rule is a rough estimation of entropy of vaporization and does not apply to all liquids. Hilderband modifies this rule above we discuss entropy changes and how we calculate the entropy changes by using the Hilderband modified Trouton’s Rule.



Leave a Reply