
Slater’s Rules give you an estimate by which to calculate the effective nuclear charge (Zeff) that an electron experiences in an atom. So why do you care? Knowing how strongly an electron feels the pull of the nucleus explains much of what you’ll care about in atoms: their size, their ionization energy, and their chemical behavior.
In an atom, the nucleus pulls on electrons, but the outer electrons don’t feel the full force of this pull because the inner electrons shield or block some of that nuclear charge. Slater’s Rules allow us to calculate how much of the nuclear charge is actually felt by a particular electron.
Why Do We Need Slater’s Rules?
The nucleus consists of protons, which provides a positive charge. The electrons have a negative charge and get attracted toward this positive charge. In a multi-electron atom, the electrons experience an attraction from the nucleus in addition to repulsion from each other. This diminishes the force of the nucleus on the outer electrons. Slater’s Rules let us apply this concept to assign a value, thereby providing us with a way of calculating the shielding effect and, therefore, the effective nuclear charge.
Key Concept: Effective Nuclear Charge (Zeff)
Before you learn Slater’s Rules, you must be familiar with Zeff, or effective nuclear charge. Zeff is the effective positive charge that an electron feels when you have taken into account the repulsion of the other electrons. You can determine Zeff using this formula:
Zeff = Z – S
Where:
Z is the number of protons, or atomic number.
S is the shielding constant obtained using Slater’s Rules.
Let’s now walk through Slater’s Rules and how you use them to get S.
How to Use Slater’s Rules
Slater’s Rules is the step-by-step procedure in the calculation to find the shielding constant. That’s how you apply Slater’s Rules. It does this by gathering electrons into several shells or groups.
Step 1: Classify the Electrons of the Atom
Classify the electrons into groups or shells of an atom according to the respective quantum number. The different groups are:
- (1s)
- (2s, 2p)
- (3s, 3p)
- (3d)
- (4s, 4p)
- (4d)
- (4f)
For example, for a nitrogen atom (with atomic number 7), the electron configuration is 1s² 2s² 2p³. We divide the electrons into two groups: (1s) and (2s, 2p).
Step 2: Assign Shielding Contributions
Once the electrons are grouped, use the following rules to assign the shielding contribution to each group of electrons:
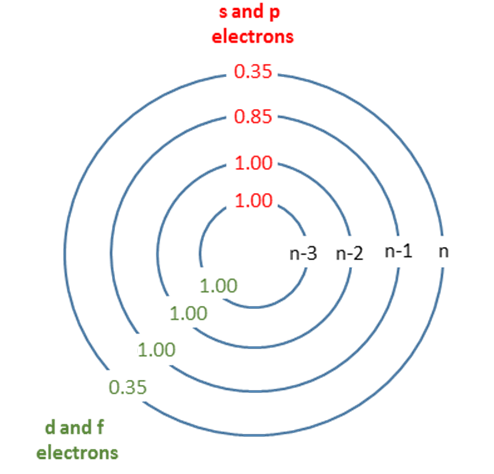
- Each adds a shielding value of 0.35 for electrons in the same group (that is, for those in the same shell as the electron of interest).
- Contributions from electrons in the group one shell below (those one shell closer to the nucleus) amount to a shielding value of 0.85.
- Electrons in groups further below (i.e., two or more shells closer to the nucleus) contribute a shielding value of 1.00.
Step 3: Calculate the Shielding Constant (S)
To get the shielding constant S sum all the contributions of other electrons according to the rules given above.
Example: Finding Zeff for Nitrogen
Calculate the effective nuclear charge, Zeff for one of the 2p electrons on a nitrogen atom.
Atomic number of nitrogen, Z = 7
Electronic configuration: 1s² 2s² 2p³
Let’s calculate S, the shielding constant:
Electrons in the same group: 2s, 2p:
There are 4 electrons in the 2s, 2p group.
Each contributes 0.35.
Total shielding by these electrons: 4 × 0.35 = 1.4
Contributions from electrons in the next lower group (1s):
There are 2 electrons in the 1s group.
Each contributes 0.85.
Total shielding by these electrons: 2 × 0.85 = 1.7
Thus, the total shielding constant (S) is:
S = 1.4 + 1.7 = 3.1
We now calculate the effective nuclear charge (Zeff):
Zeff = Z – S = 7 – 3.1 = 3.9
A 2p electron in a nitrogen atom therefore, has an effective nuclear charge of 3.9.
So, a 2p electron in a nitrogen atom experiences an effective nuclear charge of 3.9.
General Formula for Shielding Constant
Let’s summarize the process with a general formula for calculating the shielding constant (S):
S = sum (Same group) × 0.35 + sum (One shell below) × 0.85 + sum (Further shells) × 1.00
Where:
- sum (Same group) is the number of electrons in the same shell as the electron being considered.
- sum (One shell below) is the number of electrons in the shell directly below.
- sum (Further shells) is the number of electrons in shells further below.
Special Cases
Case 1: Electrons in the d and f Orbitals
Electrons in the d and f orbitals have a special rule. Electrons in the same d or f orbital group contribute 0.35, while electrons in inner shells contribute 1.00. The 0.85 factor only applies to s and p orbitals.
Case 2: Electrons in the s and p Orbitals
For s and p orbitals, we apply the same rules for calculating the shielding constant as mentioned earlier. However, when dealing with higher orbitals, the shielding becomes more pronounced due to the increased distance from the nucleus.
Visual Table for Shielding Contributions
| Shell Group | Contribution from Same Group | Contribution from One Shell Below | Contribution from Further Shells |
| s or p | 0.35 | 0.85 | 1.00 |
| d or f | 0.35 | 1.00 | 1.00 |
Examples of Slater’s Rules in Action
Example 1: Oxygen (O)
- Atomic number: 8
- Electron configuration: 1s² 2s² 2p⁴
Let’s calculate the effective nuclear charge on a 2p electron in oxygen.
- Contributions from 2s and 2p electrons: 5 × 0.35 = 1.75
- Contributions from 1s electrons: 2 × 0.85 = 1.7
Total shielding constant (S):
S = 1.75 + 1.7 = 3.45
Effective nuclear charge:
Zeff = 8 – 3.45 = 4.55
Example 2: Fluorine (F)
- Atomic number: 9
- Electron configuration: 1s² 2s² 2p⁵
Let’s calculate the effective nuclear charge on a 2p electron in fluorine.
- Contributions from 2s and 2p electrons: 6 × 0.35 = 2.1
- Contributions from 1s electrons: 2 × 0.85 = 1.7
Total shielding constant (S):
S = 2.1 + 1.7 = 3.8
Effective nuclear charge:
Zeff = 9 – 3.8 = 5.2
Conclusion
Slater’s Rules provide a simple means of estimating how much of the nuclear charge is actually experienced by an electron in an atom. It lets us group electrons and apply specific rules to their contributions to shielding so that we may calculate the effective nuclear charge and, hopefully, explain atomic behavior a little better.
Understanding Zeff is of critical importance to predicting atomic size, ionization energies, and the chemical properties of elements. These rules might seem overwhelming at first but, with practice, become indispensable for the understanding of elements’ atomic structure.


