Rydberg Equation – Derivation, Numericals.

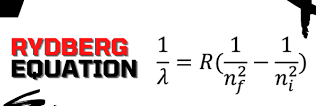
This blog will provide you with a piece of complete information about the history, derivation, and complete solved numerical problems related to the Rydberg Equation. Neil Bohr, 1913 proposed his model named Bohr’s atomic model of Max Planck. He used the concept of Planck’s quantum theory.

When an electron jumps from a higher to lower energy state or lower to higher energy orbits (perform transitions) that show atomic emission or atomic absorption spectrum because of gaining and losing of energy in hv form. The spectral lines in a particular spectrum can respond to certain wavelengths. So, in quantum mechanics, the Rydberg equation is used to calculate the wavelength and wavenumber of a spectra line.

This formula is presented by Swedish Physicist Johanness Rydberg in 1888 later on in 1913 when Neil Bohr presented his theory, he proposed the formula to calculate the radius, and energy then he calculated the Rydberg equation theoretically.

We can calculate the wavenumber as well as wavelength. Because we know that wavenumber is inversely proportional to the wavelength. Wavenumber is defined as the number of waves passing from a particular point selected and wavelength is defined as the length of a wave from the mean position to that point where there is maximum amplitude.

So, the formula derived after the derivation of the Rydberg equation is as follows;

1/λ = R(1/n₁² – 1/n₂²)

v = R(1/n₁² – 1/n₂²)

How we can derive the Rydberg equation??

Before deriving the Rydberg equation, we have to know the formula to calculate the energy and radius of the shell.

r = ε_on²h² / πme² (Radius)

E_n = -Z²me⁴ / 8ε_o²n²h² (Energy)

Let us start deriving the formula for the Rydberg equation.

E₁ = -me⁴ / 8ε_o²n₁²h² For H=1

E₂ = -me⁴ / 8ε_o²n₂²h² For H=1

∆E = -me⁴ / 8ε_o²n₂²h² – (-me⁴ / 8ε_o²n₁²h²)

∆E = hv

hv = me⁴ / 8ε_o²h² (1/n₁² – 1/n₂²)

v = me⁴ / 8ε_o²h³ (1/n₁² – 1/n₂²)

As we know,

v = cv

cv = me⁴ / 8ε_o²h³ (1/n₁² – 1/n₂²)

v = R (1/n₁² – 1/n₂²)

……….. me⁴ / 8ε_o²h³c = R …………

Here, R is Rydberg constant where R = 1.096 × 10⁷ m.

As we know that wavenumber is inversely proportional to the wavelength so the equation can be represented as:

1/λ = R (1/n₁² – 1/n₂²)

λ = wavelength of an electron

n1 = Principle quantum number for 1st shell

n2 = Principle quantum number for 2nd shell

R = Rydberg constant (1.096 × 10⁷ m^-1)

For Hydrogen

For a Hydrogen atom, there are five spectral lines. By putting the value of n we can find the inverse of wavelength called wavenumber but when we reciprocate it or divide it by unity we will find its wavelength.

The following table represents the name of the series and level of n₁ and n₂ also showing the convergence of spectral lines towards the electromagnetic spectrum.

n1	n2	Series Name	Convergence towards λ
1	2 – ∞	Lyman series	91.13 nm (Towards UV)
2	3 – ∞	Balmer series	364 nm (Towards Visible)
3	4 – ∞	Paschen Series	820 nm (Towards IR)
4	5 – ∞	Bracket series	1458 nm (Far IR)
5	6 – ∞	Pfund series	2278 nm (Far IR)

Related Numericals

• As the lowest energy series are Lyman series for the emission spectrum of Hydrogen. So, the value of n = 1. If its energy is the lowest then calculate its lowest wavelength.

We have given the value of n₁ equal to 1 and the value of n₂ is understood which is equal to 2. So, put these values in the Rydberg equation and calculate its wavelength.

1/λ = R(1/n₁² – 1/n₂²)

1/λ = 1.096 × 10⁷(1/(1)² – 1/(2)²)

1/λ = 1.096 × 10⁷(1/1 – 1/4)

1/λ = 8.228 × 10⁶ m^-1

λ = 0.1215 × 10^-4 m

• Paschen Series is also observed by the emission spectrum of hydrogen. So, it is the moderate energy state having n=3. If it has moderate energy then calculate its wavelength.

We have given the value of n₁ equal to 1 and the value of n₂ is understood which is equal to 2. So, put these values in the Rydberg equation and calculate its wavelength.

1/λ = R(1/n₁² – 1/n₂²)

1/λ = 1.096 × 10⁷(1/(3)² – 1/(4)²)

1/λ = 1.096 × 10⁷(1/9 – 1/16)

1/λ = 5.33 × 10⁵ m^-1

λ = 0.18 × 10^-4 m

TEST YOURSELF????????

• Pfund is also the emission spectrum for Hydrogen when the transition takes place from higher to lower orbit having n=5. Calculate the wavelength if the transition occurs from higher to lower orbit??

(Ans: 0.21 × 10^-3 m)

Limitations of Rydberg Equation

• Rydberg equation does not give accuracy in the results for a multi-electron system.
• As the magnitude of screening effect of inner electron for outer transition is variable so, it is impossible to compensate for it.
• So, the Rydberg formula requires some correction in it to solve these multi-electron systems. This correction is known as a quantum defect.