Lattice Energy of an Ionic Crystals

Lattice energy is a measure of the energy contained in the crystal lattice. It is the amount of energy required to separate a mole of an ionic solid into gaseous ions. Lattice energy cannot be measured empirically, but it can be calculated using electrostatic or estimated.

The lattice energy of an ionic crystal-ionic crystal is a solid consisting of positive and negative ions arranged into crystals that are made up of regularly repeated units and held together by ionic bonding and electrostatic attraction.

Ionic Crystals:

• Lattice point are occupied by possitive and negative ions.
• Hard and brittle solids.
• High melting point due to very strong electrostatic forces of attraction.
• Poor conductors of electricity in solid state but good in moten state.
• Packing of spheres depends upon the presence of charged species present and difference in the size of anions and cations.

In ionic crystals, at least two forces are operating. There are,

1. The electrostatic attractive force (Attractive potential energy)
2. Inter-atomic repulsive force (Repulsive potential energy)

The variation of the potential energy of the oppositely charged ions with their inter-nuclear separation (r) in the ionic crystal is shown below:

The total (resultant) potential energy is also shown in the same figure, r_° is the equilibrium distance at which the potential energy is minimum. Thus, if we assume that the potential energy of the ions that are separated by infinite distance, is zero, the minimum potential energy at r_° becomes numerically equal to the lattice energy U of the crystal. (At r_°, dU/dr=0)

Lattice Energy:

Lattice energy is defined as the amount of energy released when the compound ions are brought together from infinity to the bonding distances to form one mole of the solid lattice.

M⁺_(g) + X^–_(g) → MX_(s) (U= -ve)

When any system gets stability, it released energy. Minimum the energy, Maximum the stability of the system. Hence, the lattice energy is the same in magnitude but opposite in signs to the energy of dissociation of the crystal. Therefore, the greater the value of lattice energy, the greater is the energy required to decompose the ionic crystals into its ions M⁺ and X^–. Lattice energy can also be defined as the amount of energy released when gaseous cation and anion combine and form one mole of an ionic compound.

Na⁺_(g) + Cl^–_(g) → NaCl_(s)

During the formation of NaCl, both the cation and anion are in a gaseous state. When both the ions combine, they form one mole of NaCl and the amount of energy released at that time is called lattice energy.

Theoratical Calculation of Lattice Energy:

1. Born-Lande Equation:

The Born-Lande equation is a means of calculating the lattice energy of a crystalline ionic compound. In 1918, Max Born and Alfred Lande propose that the lattice energy could be derived from the electrostatic potential of the ionic lattice and a repulsive potential energy term.

Assumptions of this Model are as follows:

• Ionic crystals are constructed by a regular orientation of the oppositely charged ions which are considered as hard sphere.
• The ions are held together by the strong electrostatic forces.
• Within the crystal in addition to the coulombic forces there is a short range repulsive force originated from the interpenetration of the electronic clouds.
• At the equilibrium, the attractive and repulsive forces get mutually balanced.

Derivation of an Expression of Lattice Energy:

Let us consider an ion pair separated by a distance r and assuming that the ions are point charges. Now if these two ions are brought closer to each other then the energy of the system decreases until a minimum is reached and upon bringing the ions even closer there occurs a sharp increase in energy (as shown in the above graph). When the ions come close to each other, the only force acting first between them is the attractive force which is directly proportional to the product of charges and inversely proportional to the distance of their separation. Thus, (PE)_attraction is given by:

(PE)_attraction = − 1/4πε_° ( Z⁺Z^– e²/r)

As the ions come very close to each other, they repel one another because of the interpenetration of their electronic clouds. The (PE)_repulsion is given by:

(PE)_repulsion = B/rⁿ

Where,

• B = Repulsive constant (constant)
• n = Born exponent

In a crystal lattice, each cation/anion suffers attractive interaction from the unlike ions and repulsive interaction with the closely spaced like ions. The summation of all their geometrical interaction is referred to as the Madelung constant represented by symbol A.

(PE)_attraction = − A/4πε_° ( Z⁺Z^– e²/r) or (PE)_attraction = − A K Z⁺Z^– e²/r

The total potential energy is given:

(PE)_total = (PE)_attraction + (PE)_repulsion

(PE)_total = U = − A K Z⁺Z^– e²/r + B/rⁿ

Taking derivative both side

dU/dr = d ( − A K Z⁺Z^– e²/r ) /dr + d ( B/rⁿ ) /dr

At equilibrium, dU/dr=0

d ( – A K Z⁺Z^– e²/r ) /dr + d ( B/rⁿ ) /dr = 0

− A K Z⁺Z^– e² d (1/r) /dr + B d (1/rⁿ) /dr = 0

− A K Z⁺Z^– e² d (r^-1) /dr + B d (r^-n) /dr = 0

− A K Z⁺Z^– e² (-1) r^-2 + B (-n) r^-n-1 = 0

A K Z⁺Z^– e² / r² − Bn r^-(n+1) = 0

A K Z⁺Z^– e² / r² − Bn / r⁽ⁿ⁺¹⁾ = 0

A K Z⁺Z^– e² / r² = Bn / r⁽ⁿ⁺¹⁾

B = A K Z⁺Z^– e² r⁽ⁿ⁺¹⁾ / r² n

B = A K Z⁺Z^– e² r⁽ⁿ⁺¹⁾ r^-2 / n

B = A K Z⁺Z^– e² r^(n+1-2) / n

B = A K Z⁺Z^– e² r^(n-1) / n

Total Energy formula for 1 mole,

(PE)_total = U = N_A [− A K Z⁺Z^– e²/r + B/rⁿ ]

Put the value of B in above equation,

U = N_A [− A K Z⁺Z^– e²/r + A K Z⁺Z^– e² r^(n-1) /rⁿ n ]

U = N_A A K Z⁺Z^– e² [−1/r + r^(n-1) /rⁿ n ]

U = N_A A K Z⁺Z^– e² [−1/r + r^(n-1) r^-n / n ]

U = N_A A K Z⁺Z^– e² [−1/r + 1 / r n ]

U = −N_A A K Z⁺Z^– e² / r [ 1 − 1 / n ]

Where,

• N_A = Avogadro constant
• A = Madelung constant related to the geometry of the crystal
• Z⁺ = numeric charge number of cation
• Z^– = numeric charge number of anion
• e = charge on electron 1.602×10^-19 C
• ε_° = Permitivity of free space
• r = distance to closest ion
• n = Born exponent, typically a number between 5 to 12.

U = – N_A A K Z⁺Z^– e² / r [ 1 − 1 / n ]

• N_A = 6.023×10²³
• e = 1.6×10^-19 C
• ε_° = 8.85×10^-12 C J^-1 m^-2

By putting all these values, we get

U = – 1.39×10⁵ (Z⁺Z^– e²) / r [ 1 − 1 / n ] KJ / mol

Madelung Constant:

Madelung constant is used to determining the electrostatic potential of a single (each) ion in a crystal by approximation the ions by a point charge.

• The value of this constant depends upon the geometery of the crystal and remains independent of the value of Z⁺ , Z^– and, r.
• It is dimensionless constant.

1-D NaCl Crystal:

Electrostatic potential due to nearest ions:

U = − 2e² / 4πε_°(r_e)

Electrostatic potential due to next nearest ions:

U = + 2e² / 4πε_°(2r_e)

Electrostatic potential due to entire crystal:

U = − 2e² / 4πε_°(r_e) + 2e² / 4πε_°(2r_e) − 2e² / 4πε_°(3r_e) + ….

U = − 2e² / 4πε_°r_e (1 − 1/2 + 1/3 − 1/4 …. )

U = − 2e² / 4πε_°r_e ln2

U = − e² / 4πε_°r_e 2ln2

U = − e² / 4πε_°r_e A

A is the Madelung constant = 2 ln2

2-D NaCl Crystal:

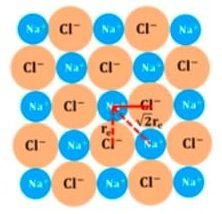
Electrostatic potential due to nearest ions:

U = − 4e² / 4πε_°(r_e)

Electrostatic potential due to next nearest ions:

U = + 4e² / 4πε_°(√2r_e)

Electrostatic potential due to entire crystal:

U = − 4e² / 4πε_°(r_e) + 4e² / 4πε_°(√2r_e) − 4e² / 4πε_°(2r_e) + 8e² / 4πε_°(2√2 r_e) ….

U = − e² / 4πε_°r_e (4 − 4/√2 + 4/2 − 8/2√2 …. )

U = − e² / 4πε_°r_e A

A is the Madelung constant = (4 − 4/√2 + 4/2 − 8/2√2 …. )

3-D NaCl Crystal:

Electrostatic potential due to nearest ions:

U = − 6e² / 4πε_°(r_e)

Electrostatic potential due to next nearest ions:

U = + 12e² / 4πε_°(√2r_e)

Electrostatic potential due to entire crystal:

U = − 6e² / 4πε_°(r_e) + 12e² / 4πε_°(√2r_e) − 8e² / 4πε_°(√3 r_e) + 6e² / 4πε_°(√4 r_e) ….

U = − e² / 4πε_°r_e (6/2 − 12/√2 + 8/√3 − 6/√4 …. )

U = − e² / 4πε_°r_e A

A is the Madelung constant = (6/2 − 12/√2 + 8/√3 − 6/√4 …. ) = 1.74756

Structure	Madelung Constant “A”
Sodium Chloride (NaCl)	1.74756
Cesium Chloride (CsCl)	1.76267
Zinc blende (ZnS)	1.63806
Wurtzite (ZnS)	1.64132
Rutile (TiO2 )	2.408
Anatase (TiO2 )	2.400
Fluorite (CaF2 )	2.51939
Corundum (Al2O3)	4.17186

Born Exponent:

Born exponent is the measure of the resistance that ions exhibit when forced to approach each other close.

1. Atoms are not hard shells and have slight compressible.
2. This factor is based on the principle quantum number of outermost electron.

Principle Quantum Number of Outermost Electron	Born Exponent “n”
1	5
2	7
3	9
4	10
5	12

What are the Born Exponent “n” for the ions listed below:

1. Li⁺
2. Br^–
3. Zn²⁺
4. O^2-

For Li⁺:

We need to determine the principal quantum number of the outermost electrons. The electronic configuration of lithium Li is 1s², 2s¹. When lithium loses its valance electron and becomes an ion Li⁺ 1s². So, that means the principal quantum number of that outermost electrons is 1 which means that Li⁺ would have a born exponent of 5.

For Br^–:

The electronic configuration of bromine Br is 1s², 2s², 2p⁶, 3s², 3p⁶, 4s², 3d¹⁰, 4p⁵. When bromine gains a electron and becomes an ion Br^– 1s², 2s², 2p⁶, 3s², 3p⁶, 4s², 3d¹⁰, 4p⁶. So, that means the principal quantum number of that outermost electrons is 4 which means that Br^– would have a born exponent of 10.

For Zn²⁺:

The electronic configuration of Zinc Zn is 1s², 2s², 2p⁶, 3s², 3p⁶, 4s², 3d¹⁰. When Zinc loses its 4s² electron and becomes an ion Zn²⁺ 1s², 2s², 2p⁶, 3s², 3p⁶, 3d¹⁰. So, that means the principal quantum number of that outermost electrons is 3 which means that Zn²⁺ would have a born exponent of 9.

For O^2-:

The electronic configuration of Oxygen O₂ is 1s², 2s², 2p⁴. When Oxygen gains two electrons and becomes an ion O^2- 1s², 2s², 2p⁶. So, that means the principal quantum number of that outermost electrons is 2 which means that O^2- would have a born exponent of 7.

What is the average Born Exponent for the salts listed below:

1. LiBr
2. ZnO

For LiBr:

For Lithium-ion, the Born exponent value is 5.

For Bromine ion, the Born exponent value is 10.

For LiBr, taking an average 5+10 / 2 means that LiBr would have a Born exponent of 7.5.

For ZnO:

For Zinc-ion, the Born exponent value is 9.

For Oxide ion, the Born exponent value is 7.

For ZnO, taking an average of 9+7 / 2 means that ZnO would have a Born exponent of 8.

2. Kapustinskii Equation:

The Kapustinskii equation calculates the lattice energy U for an ionic crystal which is experimentally difficult to determine. It is named after Anatol Fedorovich Kapustinskii who published the formula in1956.

U = – N_A A K Z⁺Z^– e² / r [ 1 − 1 / n ] J ——The Born-Lande equation

The more rigorous treatment on expression of lattice energy was done by Meyar during the calculation of repulsive potential and finally arrived at the equation:

U = – N_A A K Z⁺Z^– e² / r_° [ 1 − ρ / r_° ] J

• ρ = 34.5 pm

For the cases where lattice type is unknown Madelung constant is not available, we can’t use the Born-Lande equation to determine the lattice energy of such compounds and for such lattices, the Kapustinskii equation is used.

U = – 1.2×10⁵ x Z⁺Z^– / r_° [ 1 − 34.5 / r_° ] KJ / mol

Where

• X= Total number of ions in the formula of ionic compounds (2 for NaCl, 3 for MgF₂ etc)
• It incrases with incraesing charge on the ions
• It is larger for smaller ions

Hess’s Law of Constant Heat Summation:

Born Haber’s process is based upon Hess’s law. This law was discovered experimentally in 1840 by German Henry Hess, a professor of St. Petersburg, Russia.

Statement:

“The amount of heat evolved or absorbed during a change (physical or chemical) remains the same, whether the change takes place in one or several steps”

Let us consider A can be converted into D directly in a single step with enthalpy change ∆H, if the reaction can have a route from A B C with enthalpy changes ∆H₁, ∆H₂, ∆H₃ respectively. According to Hess’s Law,

∆H = ∆H₁ + ∆H₂ + ∆H₃

Verification of Hess’s Law:

The formation of sodium carbonate can be studied as a single step process or in two steps process.

Single Step Process:

2NaOH + CO₂ → Na₂CO₃ + H₂O ∆H = −89.08 KJ/mol

Two Step Process:

NaOH + CO₂ → NaHCO₃ ∆H = −48.06 KJ/mol

NaHCO₃ + NaOH → Na₂CO₃ + H₂O ∆H = −41.02 KJ/mol

According to Hess’s law,

∆H = ∆H₁ + ∆H₂

−89.08 = −48.06 − 41.02

−89.08 = −89.08

Hence Hess’s law is verified

Application of Hess’s Law:

There are many compounds for which ∆H cannot be measured directly by the calorimetric method.

1. Heat of formation of CCl₄ because it cannot be prepared by the direct reaction of carbon and chlorine. Similarly, Heat of formation of boron oxide (B₂O₃) and aluminium oxide (Al₂O₃) provide problems for the measurment of standard enthalpies of their formation.
2. Heat of formation of carbon monoxide due to th formation of carbon dioxide. Therefore, the enthalpy of such compounds can be measured indirectly by Hess’s law.

C (graphite) + O₂ → CO₂ ∆H (graphite) = −393 KJ/mol

CO + 1/2 O₂ → CO₂ ∆H₂ = −283 KJ/mol

C (graphite) + 1/2 O₂ → CO ∆H₁ = ? KJ/mol

According to Hess’s law,

∆H = ∆H₁ + ∆H₂

∆H₁ = ∆H − ∆H₂

∆H₁ = −393 − (−283)

∆H₁ = −110 KJ/mol

Born Haber Cycle-To Find Lattice Energy:

Born Haber cycle is the special application of Hess’s law which is used to determine the lattice energy of binary ionic compounds. In the Born Haber cycle, there are many terms of energy is involved during the process.

Heat of Formation:

“The amount of heat released when 1 mole of a compound is formed from its elements in the pure and stable state”

Na_(s) + 1/2 Cl_2(g) → NaCl_(s) ∆H_f=?

The difference in lattice energy and heat of formation is that in lattice energy gaseous cation and anion combine and form 1 mole of an ionic compound but in heat of formation pure and stable state of an atom combines and forms an ionic compound.

Realation between ∆H_f and Lattice Energy:

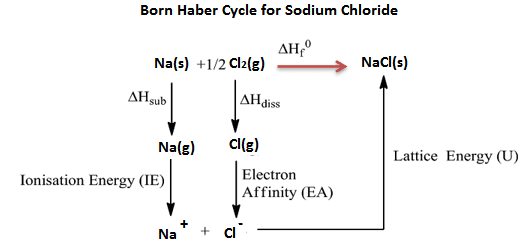
1. In the formation of NaCl, two methods are used. One is direct method (single step) and other is indirect method (several steps).
2. The indirect method is used to find the lattice energy of NaCl (ionic bond) is Born Haber cycle.
3. The energy released in single step is called ∆H_f
4. The energy released in several steps is called Lattice energy.
5. The principle of Born Haber cycle is Hess’s law (energy involve in single step = energy involved in different steps)

∆H_f = ∆H_sub + I.E + ∆H_diss + E.A + U

Now we calculate the lattice energy of sodium chloride,

In Single Step:

Na_(s) + 1/2 Cl_2(g) → NaCl_(s) ∆H_f = -411 KJ/mol

In Several Steps”

Na_(s) → Na_(g) ∆H_sub = +108 KJ/mol

Na_(g) → Na⁺_(g) I.E = +496 KJ/mol

1/2 Cl_2(g) → Cl_(g) ∆H_diss = +122 KJ/mol

Cl_(g) → Cl^–_(g) E.A = -349 KJ/mol

Na⁺_(g) + Cl^–_(g) → NaCl_(s) L.E = ?

∆H_f = ∆H_sub + I.E + ∆H_diss + E.A + U

-411 = +108 + 496 + 122 – 349 + U

U = = -411 -108 -496 -122 +349

U = – 788 KJ/mol

The lattice energy U is -788 KJ/mol

In the case of MgO,

The first electron affinity during the formation of O^– is negative because it is exothermic and heat is evolved. But second electron affinity is positive because the second electron is repelled by an already present electron that’s why the energy is given by the adding electron. So, the second electron affinity is endothermic and positive.

∆H_f = ∆H_sub + I.E₁ + I.E₂ + ∆H_diss + E.A₁ + E.A₂ + U

Lattice Energy For Some Ionic Compounds:

Compounds	Lattice Energy (KJ/mol)
LiF	1030
LiCl	834
LiI	730
NaF	910
NaCl	788
NaBr	732
NaI	682
KF	808
KCl	701
KBr	671
CsCl	657
CsI	600
MgCl2	2526
SrCl2	2127
MgO	3795
CaO	3414
SrO	3217
ScN	7547

Factor On Which Lattice Energy Depends:

Na⁺_(g) + Cl^–_(g) → NaCl_(s) (L.E)

• Energy is released due to attraction between Na⁺ and Cl^–
• More the attraction = more the bond strength
• More the energy released = more the stability

F ∝ q₁q₂/ r²

F = K q₁q₂/ r²

• q₁ and q₂ is charges on ions.
• r is the distance between them.

F ∝ Lattice energy

Factor 1:

Lattice Energy ∝ Z⁺Z^–

Lattice energy is directly proportional to the charge on cation and anion.

Factor 2:

Lattice energy ∝ 1/size

Lattice energy is inversely proportional to the size of the cation and anion.

Which one has highest Lattice energy?

a) NaF

b) MgF₂

c) AlF₃

• Anion is same in given compounds.
• Cation is different so, we see only the charge factor not the size factor because from left to right size is slightly increase. The charge on Na, Mg, Al is +1, +2, +3 respectively. As, greater the charge, greater the lattice energy.

Order:

NaF < MgF₂ < AlF₃

Which one has highest Lattice energy?

a) Na₂O

b) MgO

c) Al₂O₃

• Anion is same in the given compounds.
• Cation is different so, we see only the charge facyor not the size factor. The charge on Na, Mg, Al is +1, +2, +3 respectively.

Order:

Na₂O < MgO < Al₂O₃

Which one has highest Lattice energy?

a) Li₂O

b) Li₃N

• Cation is same in given compounds.
• Anion is different so, we see only the charge factor not the size because N and O is very closed to each other. The charge on O and N is -2 and -3 respectively.

Order:

Li₂O < Li₃N

Which one has highest Lattice energy?

a) NaCl

b) KCl

• Anion is same in given compounds.
• Cation is different so, we see only the charge size factor not the charge because both the Na and K has +1 charge. The size of K is larger than Na. (greater the size, smaller the lattice energy)

Order:

NaCl > KCl

Which one has highest Lattice energy?

a) NaF

b) NaCl

c) NaBr

• Cation is same in given compounds.
• Anion is different so, we see only the size factor not the charge factor because F, Cl, Br have -1 charge. As we move from down the group in the peruidic table size increases.

Order:

NaF > NaCl > NaBr

Which one has highest Lattice energy?

a) NaF

b) MgCl₂

• Both the cation and anion is different.
• Compare the cation Na⁺ < Mg²⁺ (charge factor)
• Compare the anion F^– < Cl^– (size factor)

if both the size and charge factor fight with each other then the charge factor will be dominant over the size.

Order:

NaF < MgCl₂