
Planck’s quantum Theory
Understanding a complete notation of Bohr’s Radius and the Bohr Atomic model is necessary to explain some of the postulates of Planck’s quantum theory. Therefore, Planck’s Quantum theory is given by Max Plancks. Max Plancks proposed the quantum theory in 1900 to explain the emission and absorption of radiations, and he also proved that electron has a wave nature. The main points of the theory are:
- Energy is not emitted or absorbed continuously. Instead, it is emitted or absorbed discontinuously and in the form of wave packets called photons. Each wave packet or quantum is associated with a definite amount of energy. Hence, in the case of light, the quantum of energy is often called the photon.
- The amount of energy associated with the quantum of radiation is directly proportional to the frequency of radiation. Therefore, the relation becomes:
E ∝ v
E = hv
In this equation, “h” is Planck’s constant and has the value of 6.626×10-24 Js.
- A body can emit or absorb energy in the form of quanta.
E = hv
v = c / λ
E = hc / λ
Bohr’s Atomic Model
Radius is the distance from the nucleus to the outermost shell. Derivation of Bohr’s radius involves the complete knowledge of Bohr’s Atomic theory. So, it is necessary to have a piece of complete knowledge of Bohr’s Atomic Theory. Bohr’s Atomic Theory is a conceptual study about momentum, energy, radius, frequency, and wavenumber in orbit. Bohr’s Atomic model is based on Max Planck’s Theory.
Bohr’s atomic model was presented in 1913 by Neil Bohr. According to the Rutherford Atomic model, its classical theory proposed that due to attraction between the electrons and nucleus, electrons will fall into the nucleus and the atom should collapse. He didn’t give any concept of the orbits or shells. He gave the spiral concept of the electron and nucleus.
To cure this remedy Neil Bohr presented a model in which he modified the Rutherford Atomic model and gave the concept of orbits and shells that have fixed energy and size. Therefore, Bohr explains the stability of an atom. He also concluded that energy and radius have direct relation i.e. the orbit close to the nucleus has low energy and have a less atomic radius but on the other hand, the orbits far away from the nucleus have high energy and greater atomic radius.
So, it is concluded that Bohr’s Atomic model was the improvement made in the earlier cubic meter, plum-pudding theory as well as Rutherford’s Atomic Model.
Postulates of Bohr’s Atomic Model
Bohr’s Atomic Model is based on the Quantum theory of Max Plancks. Following are the postulates of Bohr’s Atomic Model.
- Electrons revolve in one of the circular orbits outside the nucleus. Each orbit has fixed energy and the quantum number is assigned to it.
- Electrons present in a specific and particular orbit can never emit or absorb energy. It can emit or absorb energy when they jump or come back from one orbit to another orbit. So, excitation or de-excitation of electrons results in the absorbance or emittance of the energy.
- When an electron jumps, the energy change ∆E is given by Planck’s equation. In the given equation, ∆E is the energy difference between any two orbits with energies E1 & E2.
∆E = E2 – E1 = hv
- Electrons can revolve only in those orbits having a fixed angular momentum (mvr). The electron is inbound to remain in one of these orbits and not in between them. So, angular momentum is quantized. By this formula, the primitive values for angular momentum are, therefore, h/2π, 2h/2π, and 3h/2π.
mvr = nh / 2π (where n = 1, 2, 3……………..)
Limitations of Bohr’s Model
Following are the demerits or limitations of Bohr’s Atomic Model. These demerits are written as under:
- Neil Bohr does not explain the Zeeman effect. Zeeman effect is the magnetic effect on the spectra of atoms.
- Neil Bohr also failed to explain the stark effect. The Stark effect is the electrical effect on the spectra of atoms.
- It violates the Heisenberg uncertainty principle. It states that it is difficult to explain the momentum and position of an electron at the same time.
- These concepts avoid explaining the spectra obtained from the larger atoms.
Bohr’s radius by Bohr’s Atomic Model
Radius is defined as the distance from the nucleus to the outermost shell of an atom is called Radius. Bohr’s Radius is the physical term in atomic physics. It explains the most probable distance between the nucleus and the electron present at the ground state. Bohr explains the radius of that atom comprises only one orbit and one electron just like hydrogen. So, the derivation of radius for a genera atom is given below: we have to derive the following equation:
r = (∈o h2 / π m e2) n2
For a general atom, consider an electron of charge ‘e’ revolving around the nucleus having the effective nuclear charge Ze+. Let, the mass of electron = m, the velocity of a revolving electron = v, and the radius of orbit = r. According to Columb’s law: “There is the electrostatic force of attraction between oppositely charged particles.” As we all know that there is a positive charge on the nucleus and a negative charge on the electron. So, an electrostatic force of attraction is always developed.
So, when an electron is moving in a circular orbit, it moves because it possesses centripetal force. Centripetal force is defined as: The force which allows an object or an electron to move in a circular orbit is called centripetal force.
Fc = mv2/r ……(1)
So, electrostatic force of attraction is given by:
Electrostatic force = Ze2/4π∈or2 ……..(2)
Where ∈o is the relative permittivity and its value is 8.84 × 10-12 C2J-1m-1.
By equating or comparing equation 1 with 2 that means right-hand sides are equal so on this behalf left-hand sides are also equal. So, by comparing both equations:
mv2/r = Ze2/4π∈or2
mv2 = Ze2r/4π∈or2
mv2 = Ze2/4π∈or………(3)
BY REARRANGING THE ABOVE EQUATION (3), it becomes:
r = Ze2/4π∈omv2………..(4)
- According to equation (4), the radius of the moving electron is inversely proportional to the square of its velocity.
- However, it also suggested that or concluded that the electrons should move faster nearer to the nucleus in an orbit of a small radius.
- Therefore, it also gives the brief idea that, if a hydrogen atom has many possible orbits, then the promotion of electrons to higher orbits makes it move with less velocity.
In order to explain the factor of velocity from the equation (4), we use Bohr’s postulate number 4. He discussed the angular momentum of electrons. Angular momentum is the momentum of a circular orbit. It is given by:
mvr = nh / 2π
v = nh / 2πmr
By taking square on both sides.
v2 = n2h2 / 4π2m2r2 ………………(5)
By putting the value of v2 from equation 5 in equation 4.
r = Ze2/4π∈om (n2h2 / 4π2m2r2)
r = Ze24π2m2r2/4π∈om n2h2
= Ze2πmr/∈o n2h2
r = ∈o n2h2/Ze2πm ………….(6)
For hydrogen atom Z = 1, the equation for the radius of the hydrogen atom is:
r = ∈o n2h2/Ze2πm = (∈o h2/Ze2πm)n2 ……………(7)
According to equation number 7, the radius of a hydrogen atom is directly proportional to the square of a number of orbits. So, the higher the orbit has the higher the radius, and vice versa. The collection of parameters (∈o h2/Ze2πm) is a constant factor. So,
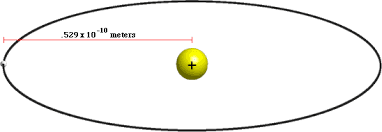
(∈o h2/Ze2πm) = 0.529Å = 0.529 × 10-10 m
BY PUTTING THE VALUES OF THE CONSTANT FACTOR IN EQUATION (7).
r = 0.529Å × n2
Conclusion for Bohr’s Radius
- By putting the value of “n” as 1, 2, 3, 4 ……….. the radii of orbits of the hydrogen atom are:
| n = 1 | r1 = 0.529Å |
| n = 2 | r2 = 2.11 Å |
| n = 3 | r3 = 4.75 Å |
| n = 4 | r4= 8.4 Å |
| n = 5 | r5 = 13.22 Å |
- The comparison between these radii shows that the distance between orbits goes on increasing as we move from the 1st orbit to the higher orbit. The orbits are not equally spaced.
- The second orbit is four times away from the nucleus the first orbit, the third orbit is nine times away, and similarly furth orbit is sixteen times away.
Uses of Bohr’s Radius
However, Bohr’s radius is not used in physical terms but is highly used because of its promising presence in calculations of other fundamental physical contents. These physical contents may include Atomic units, and the fine structure constant.



Leave a Reply