
Arrhenius equation tells the effect of temperature on the rate of reaction. Basically, by changing temperature how to rate constant is changed. If the rate constant is changed, the rate of reaction is also changed. We studied the relation between temperature and rate constant.
In most of the reactions, on increasing temperature, the rate also increases or rate constant increases.
Rate = K[conc]n
Rate ∝ K (K is rate constant)
Here we discussed two methods between rate constant and temperature.
- Approx dependency
- Arrhenius equation
Approx Dependency of “K” on “T”
Generally, on a 10°C rises in temperature, the rate constant (ROR) nearly doubles.
Temperature Coefficient
The temperature coefficient is the ratio of two rates constant.
Temp coefficient = K(t+10°C) / Kt°C
Most of the time, the value of the temperature coefficient is between 2 and 3. For example, the value of the temperature coefficient is 3.
| 10°C | 20°C | 30°C | 40°C |
| K | 3K | 9K | 27K |
For a Particular Reaction, the temperature coefficient is 2. If the rate of reaction at 20°C is “x”. Find the rate at 80°C?
The temperature coefficient of 2 means temperature increases by 10°C the rate becomes double.
| Temp | 20°C | 30°C | 40°C | 50°C | 60°C | 70°C | 80°C |
| Rate | x | 2x | 4x | 8x | 16x | 32x | 64x |
So at 80°C, the rate is 64x.
Arrhenius Equation
Arrhenius equation is the accurate dependency of “K” on “T”. According to Arrhenius equation:
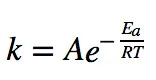
- K = Rate constant
- A = Frequency factor or Arrhenius factor or pre-exponential factor
- e = exponential constant
- Ea = Activation energy (J/mol)
- R = General gas constant (8.314 L/mol)
- T = Temperature (Kelvin)
Graphy Between Rate Constant “K” and Temperature “K”

What is meant by the Collision Theory?
According to collision theory, molecules effectively collide and give a product. The word effectively means at a proper angle and at proper energy or particular energy. Generally, in one second, in one cm3 volume, 1027 collisions were done. But all the collisions between reactant molecules are not effective (gives product).
For Effective Collision
Orientation Barrier

Energy Barrier
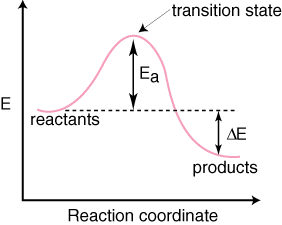
Activation energy, Ea is the minimum amount of energy that should be given to reactant molecules already having some energy so that they can cross the energy barrier.
Graph: Number of Molecules vs Kinetic Energy
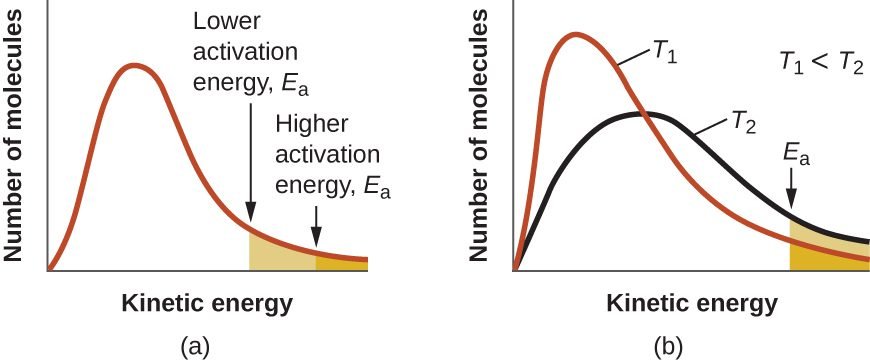
- Number of molecules that cross the energy barrier = e-Ea/RT
- By increasing the temperature, the number or fraction of molecules increases.
F.O.M which cross activation energy = e-Ea/RT
- Higher the e-Ea/RT, the higher the rate
Rate ∝ e-Ea/RT
K ∝ e-Ea/RT
K = A.e-Ea/RT
The above equation is the Arrhenius equation.
Logarithmic Form of Arrhenius Equation OR Method to Find Activation Energy
K = A.e-Ea/RT
Taking natural log on both side
ln K = ln (A.e-Ea/RT)
ln K = ln A + ln e-Ea/RT
ln K = ln A – Ea/RT ln e
ln K = ln A – Ea/RT (1)
ln K = ln A – Ea/RT
Graph: ln K vs 1/T

How We Find the Activation Energy?
We find the activation energy by using the Arrhenius equation. We can find different values of rate constant for different values of temperature then we plot the graph, we will find the slope and equated to -Ea/R.
Slope = tanθ = -Ea/R
Arrhenius Equation For Log base 10 (log10)
K = A.e-Ea/RT
ln K = ln (A.e-Ea/RT)
ln K = ln A + ln e-Ea/RT
ln K = ln A – Ea/RT ln e
ln K = ln A – Ea/RT (1)
ln K = ln A – Ea/RT
2.303 log10K = 2.303 log10A – Ea/RT
Divided by 2.303 on both side
log10K = log10A – Ea/2.303RT
log K = log A – Ea/2.303RT
The above equation is the logarithmic form of the Arrhenius equation.
Graph: log K vs 1/T
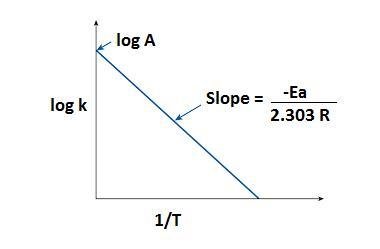
How We Find the Activation Energy?
We can plot a graph between log K vs 1/T and slope of the graph is -Ea/2.303R, we know the value of R (8.314) and we can find the activation energy.
Numerical
For nth order reaction, the frequency factor A is 100 and activation energy is 19137 J/mol. Find the rate constant at 727°C?
t = 727°C + 273 = 1000K
log K = log A – Ea/2.303RT
Both “A” and “Ea” is temperature independent
log K = log 100 – 19137/2.303×8.314×1000
log K = log 100 – 19137/ 19137
log K = log 102 – 1
log K = 2log 10 – 1
log K = 2-1
log K = 1
K = 101



Leave a Reply